Mathematicians' Latest Feat Outdone by "Vampire Einstein" Tile

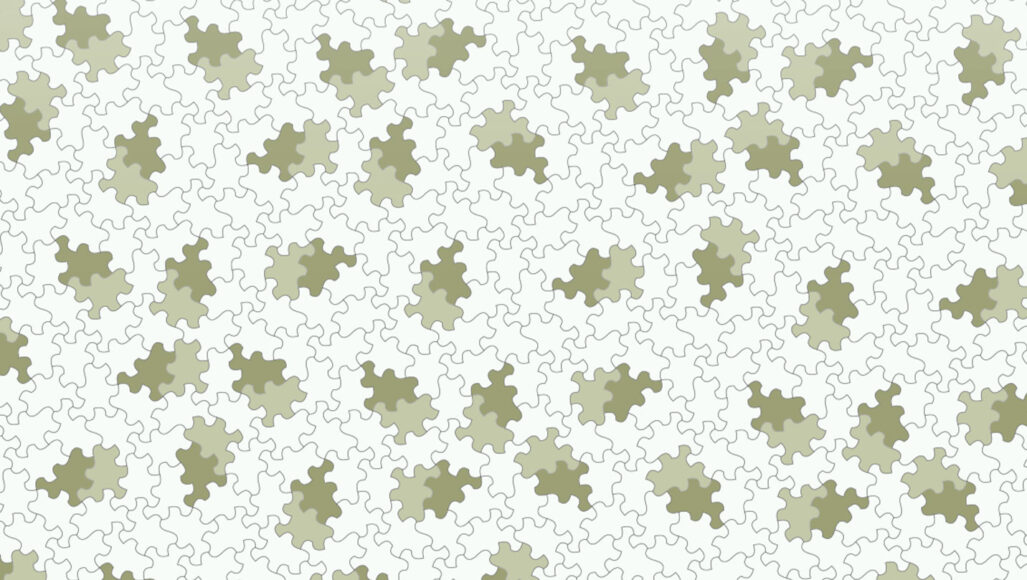
Einstein was just introduced to its vampire counterpart.

A few months ago, researchers announced the discovery of the first “einstein” - a tile that can cover an infinite plane but only in a pattern that never repeats. (source) The same team has now found a shape that's even more unique. The original einstein (nicknamed “the hat”) made a pattern that included both the hat and its mirror image. In contrast, the new tile creates a pattern that never repeats but does not involve reflections - the researchers posted this on arXiv.org on May 28th. Due to its lack of reflection, it has since been named the "vampire einstein." (It bears no connection to the physicist; the term einstein translates to "one stone" in German.) This discovery forms a part of a group of vampire einsteins, which the scientists dubbed "spectres."

Mathematicians have long been fascinated by the way tiles cover an infinite plane without overlaps or gaps between them. While other tiles can be arranged to form a non-repeating pattern, einsteins are unique in that this is the only way they can tile. In the past, mathematicians knew of certain sets of tiles that could tile a plane with a non-repeating pattern but only this year became aware of a tile that could do it solo.

After discovering the first einstein tile, the researchers wondered if it was possible to find a tile that would create a non-repeating pattern without any reflected versions. Starting from a form similar to the hat and curving its edges, the reflection of this new tile no longer fits together, resulting in a vampire einstein tile.

“I would never have predicted that we’d stumble upon a shape that solves this [vampire einstein] subproblem so quickly,” stated Craig Kaplan, a computer scientist at the University of Waterloo in Canada.

Our objective is to provide precise and engaging science news to the public. Our mission has never been more vital than it is today. As a non-profit news organization, we rely on your generosity. Your contribution allows us to keep our content available and free for the upcoming generation of scientists and engineers. Please make a donation today to invest in quality science journalism.