"This Complex Maze Reveals Connections on Quasicrystal Surfaces"

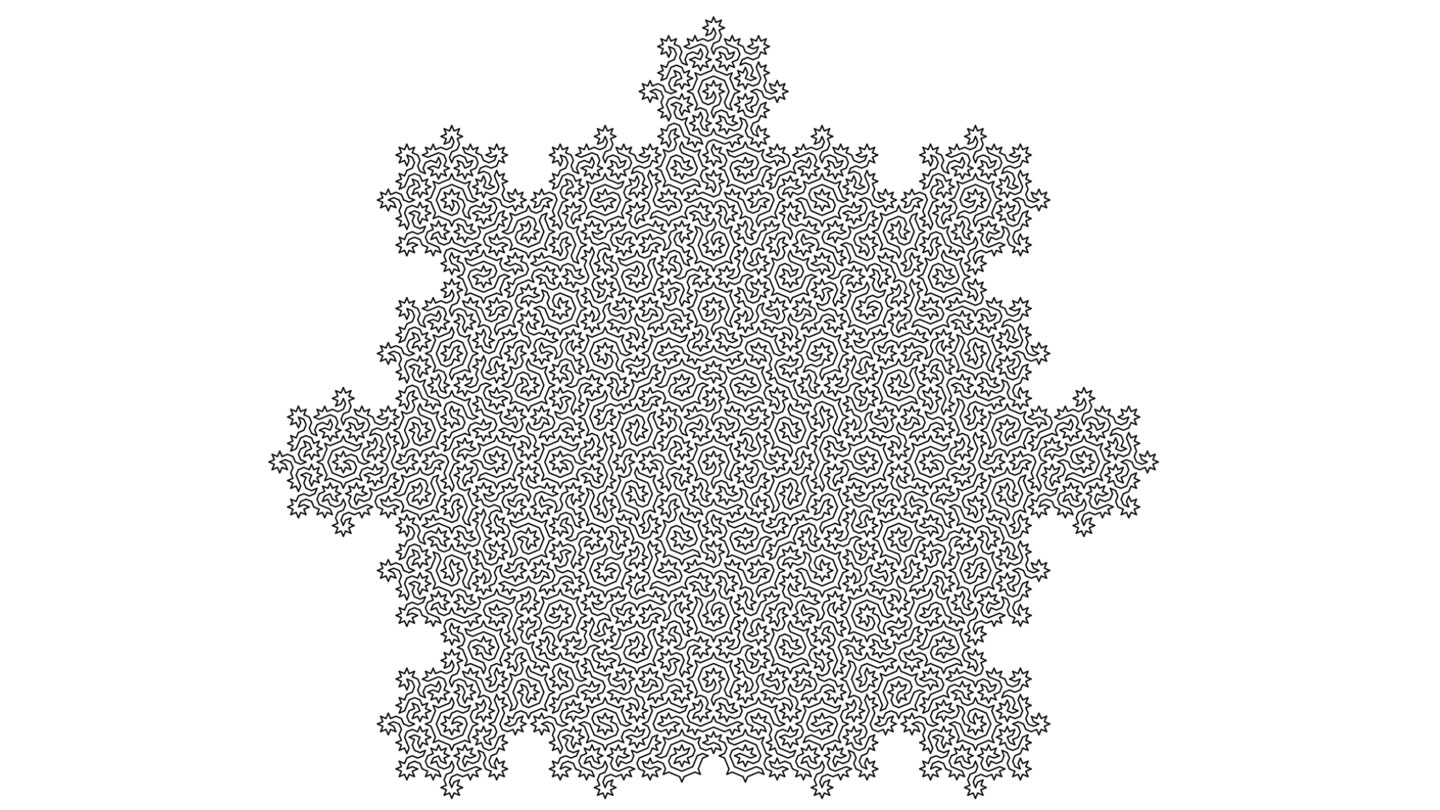
This maze of jagged curls looks like something out of the world’s hardest puzzle book. How fast do you think you can solve it?

Stuck? Don’t worry. It’s actually more of a connect the dots puzzle.

The labyrinthine black path is the shortest nonintersecting route to connect every point on a kaleidoscopic, “quasicrystalline” surface, researchers report July 10 in Physical Review X.

Shobhna Singh, a theoretical physicist at Cardiff University in Wales and her colleagues examined a type of pattern known as an Ammann-Beenker tiling, which fills a two-dimensional space using square- and rhombus-shaped tiles. Like some kaleidoscope images, Amman-Beenker tilings are organized but the pattern doesn’t repeat itself regularly. The atoms in certain types of quasicrystals — ordered but nonrepeating chemical structures — adopt a similar geometry (SN: 10/5/11).

The researchers found a path that touches on every vertex in an Amman-Beenker tiling, without crossing itself, before ending back where it started. Called Hamiltonian cycles, these pathways form a closed loop that you can trace without picking up your finger.

Solving a Hamiltonian cycle for even one type of tiling is no small feat. But this particular cycle — and possibly others — could help address scientific challenges. For example, it could make certain quasicrystals more efficient catalysts, substances that reduce the energy required for a chemical reaction. In theory, if molecules involved in the reaction arranged themselves along the Hamiltonian path of such a quasicrystal, they could attach to the surface with maximum efficiency.

Moving forward, the team will search for Hamiltonian cycles on other types of tilings, Singh says. They’re also looking for new ways to apply their Hamiltonian cycle to existing challenges. “The most interesting application could be one which we have not thought about.”